Page 12 - Application Guide Semiconductor Fuse Link

Estimation of fuse life under cyclic overloads

Section 2 described the use of the factor A’2 , which is applied to the continuous current rating of the
fuse to allow for the effect of cyclic or non-continuous currents.If the duty cycle contains «overloads»
(periods when the current is in excess of the fuse current rating), it is necessary to consider their
magnitude and duration, in relation to the time-current characteristic of the fuse. Consider the simple
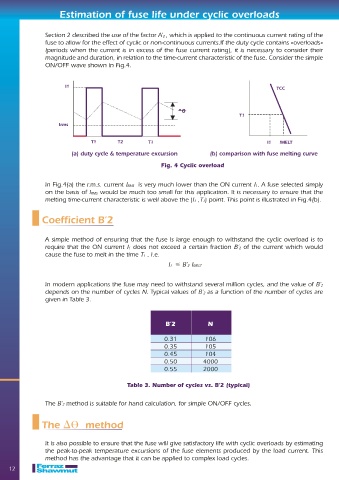
ON/OFF wave shown in Fig.4.

I1
TCC

T1
Irms

T1 T2 T1 I1 IMELT
(a) duty cycle & temperature excursion (b) comparison with fuse melting curve

Fig. 4 Cyclic overload

In Fig.4(a) the r.m.s. current IRMS is very much lower than the ON current I1. A fuse selected simply
on the basis of IRMS would be much too small for this application. It is necessary to ensure that the
melting time-current characteristic is well above the (I1 ,T1) point. This point is illustrated in Fig.4(b).

Coeffi cient B’2

A simple method of ensuring that the fuse is large enough to withstand the cyclic overload is to
require that the ON current I1 does not exceed a certain fraction B’2 of the current which would
cause the fuse to melt in the time T1 , i.e.

I1 B’2 IMELT

In modern applications the fuse may need to withstand several million cycles, and the value of B’2
depends on the number of cycles N. Typical values of B’2 as a function of the number of cycles are
given in Table 3.

B’2 N

0.31 106
0.35 105
0.45 104
0.50 4000
0.55 2000

Table 3. Number of cycles vs. B’2 (typical)

The B’2 method is suitable for hand calculation, for simple ON/OFF cycles.

The method

It is also possible to ensure that the fuse will give satisfactory life with cyclic overloads by estimating
the peak-to-peak temperature excursions of the fuse elements produced by the load current. This
method has the advantage that it can be applied to complex load cycles.
12